Membranes and Shells
Supplement for chapter 15
Another great contribution by Prof. Jim Woodhouse here; it applies more broadly than this chapter, but this is as good as any.
Membranes vs. Shells
Membranes are thin enough that waves like sound waves in air travel across them, in that all wavelengths travel the same speed. Shells are thicker, and almost always have shorter wavelengths traveling faster. This is because the increased force opposing bending, force that gets bigger (and therefore more restoring force) as the curvature gets bigger. Short wavelengths have bigger curvatures because they are quickly oscillating up and down.
Traveling deformation of a thin stretched membrane, rigidly fixed at its boundaries, after being kicked suddenly upward at the red spot. White represents velocity perpendicular to the surface upward, i.e. in a direction toward the reader, black encodes downward velocity away from the reader. Note that when the border is encountered, the reflected wave leads with black if it approached with white leading. This shows that the wave reflects with a sign reversal, ensuring that the border itself does not move. That is, at the border the incoming wave meets the counter propagating reflected wave, and the two just cancel there.
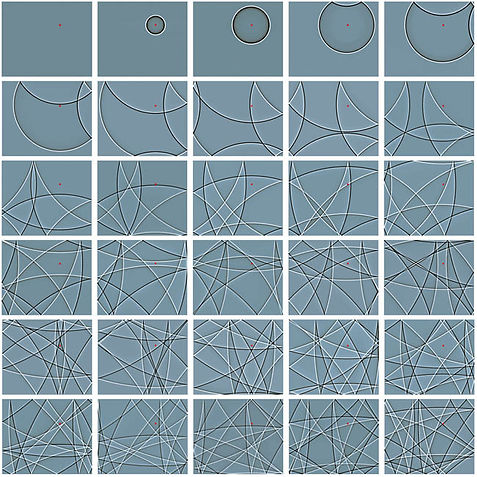
If you start the disturbance at the red dot and subsequently "listen" there, velocity spikes arrive as echoes; the first few spikes and the waves responsible for them are shown below; look carefully for the red dot on the upper right quadrant (it stays fixed, as a microphone position).

String networks
New types of stringed instruments are possible using networks of tension strings; visit here for reference to the Tritare; there is a fairly complex network at * dead link * http://www.acoustics.org/press/151st/Leger-6.wav
Paul Falstad's Rectangular Membrane (try *dead link* this version for sound)
(some browsers might not give sound, even if Java 2 or higher is installed).
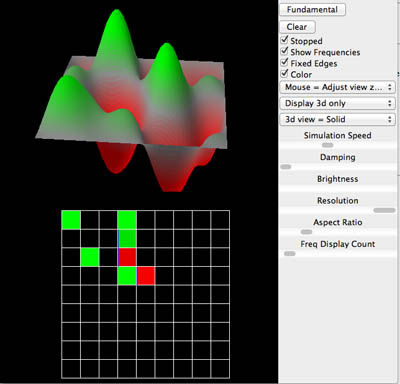
This Java applet is well poised to examine either the sound in a two dimensional enclosure filled with air, or the displacement of a membrane. In the former case, leave "Fixed edges" unchecked, in that case the amplitude shown at the top is the pressure in the room. In the latter case, check fixed edges and the amplitude becomes that of a stretched membrane locked down on its perimeter. Square or rectangular shaped can be investigated.
The initial wave can be set by "poking", "striking", or "shaping" with the mouse, or set by placing the mouse in the boxes at the bottom (if "Show frequencies is checked") and setting the amplitude and phase of the modes corresponding to the box. If fixed edges is checked, the upper left box controls the amount of the nodeless lowest mode. By experimenting after pressing "Clear" each time, you can place the mouse over other boxes, click and adjust the amount of other modes that have more and more nodes (in x or y), depending on how far the box is from the upper left corner.
Chladni Patterns
It is worth noting that the metal sheet plates, such as those on the videos here, are often driven by sinusoidal motor supplying up and down motion to the center of the plate, via a bolt there. Therefore, we must expect that not all the modes of the plate (if it were free of the bolt) will be excited; any mode with a nodal line passing through the center is not excited since such modes cannot respond where they have not amplitude. Note that this is true for all the modes seen here - none have nodal line passing through the center. (Sand often collects in a spot near the bolt for a different reason - this is not a "nodal line").
* Image missing in live site *
The patterns below are particularly nice, but there is no sound. Can you tell whether the driving frequency is rising or falling with time anyway?
* Image missing in live site *
Bells
Bells are shells of various shapes, designed to oscillate at a pleasing set of frequencies, a set that gives a holistic bell tone.
Terhardt's classic paper on the strike pitch of bells. A little technical but OK if you are doing a project on bells. He doesn't seem to know about or else is a non-believer in the autocorrelation theory of pitch.
New types of bells continue to be invented, in fact, there is a resurgence made possible by computer design. See e.g. www.ausbell.com.au.
Bell Plates
University of New South Wales web site with much information about bell plates. Many example sound files of plate cut different ways can be found here, including a demonstration of the dependence of the tone of the bells on shape that ought to get a web award.
Accessible article on bell plates: Lavan, D., Hogg, S. and Wolfe, J. (2003)
"Why do bell plates ring?" Acoustics Australia, 31, 55-58.
Rectangular bell plates:
* Dead Link* http://products.kolberg-percussion.com/en_GB/363/category.html
Paul Matisse's bells
Anyone visiting Boston should consider seeing and hearing Paul Matisse's installations at the Kendall - MIT "T" station. They are interactive! A full description is * Dead Link* here.
A two-dimensional spring array - PHET
The PHET project at the University of Colorado, Boulder provides this simulation of a *!target requiers Flash-Player* planar 2D array of masses and springs. The number of masses may be adjusted from one to 100, and the masses can be "plucked" or normal modes excited individually or in combinaiton. This is not a stretched array of beads and strings; rather, it is a set of masses connected by springs. At rest, there is no tension and the interactions result from changing the distance between adjacent masses, which causes them to push apart or pull together. Again, normal modes of different frequency appear.
There is unfortunately no information about frequency or power spectra. However, you can for example resonantly pump the lattice by oscillating the mouse; you can pluck a mass and see a wave heading out from there, and many more variations. This model is closest to the in-plane (not deforming out of plane) compression waves traveling along a solid surface.
